Twisted Logic: The Story of the Paradox
Origin and meaning
The origin of the word paradox comes from the Latin paradoxis, which in turn comes from the Greek paradoxos, which roughly translates into "aside belief". Paradoxes are often presented in the form of a statement or a question. A couple of the best-known paradoxes include problems dealing with self-reference, confusing levels of reasoning and false statements.
 Zeno’s famous paradox
Zeno’s famous paradox
Achilles and the tortoise
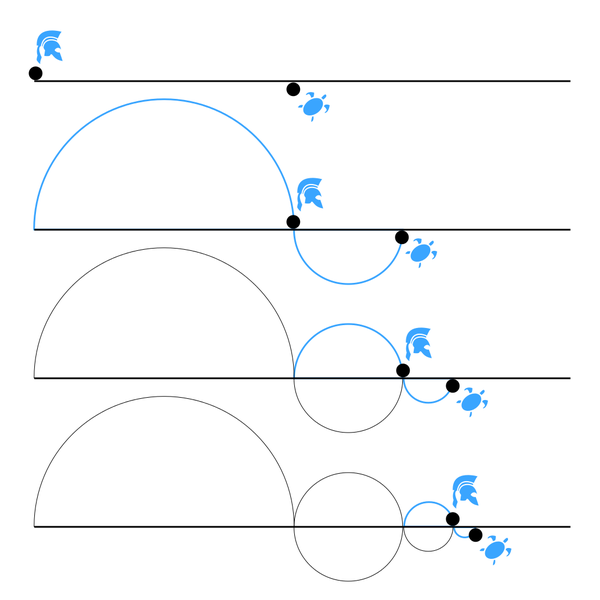
Since ancient times, people have been attempting to find, invent and explain different paradoxes and how they relate to the physical world. One of the many paradoxes attributed to the ancient Greek philosopher Zeno classically involves a race between the champion Achilles and a tortoise. Confident in his superior athletic abilities, Achilles gives the tortoise a head start of 100 meters.
The race
So the race starts, with both participants running at different constant speeds (one fast, one slow, but with no acceleration). After some period of finite time has passed, Achilles will have covered the 100 meters to where the tortoise started the race from, but during this time, the tortoise “running” at his slower pace will also advance some small distance, let’s say 3 meters. It then takes Achilles another period of finite time to run these 3 meters. However, during this period, the tortoise has advanced another small distance. Thus, whenever Achilles reaches somewhere the tortoise has been, he still has farther to go to catch him. If the cycle is repeated many times, Achilles will get very close to the tortoise, but will never catch him in the race, let alone pass him. This defies common sense and leads to a paradox. It is intuitive that a faster runner can overtake a slower one; however, presented as it is above, it seems impossible to do so.
How do we think about this problem and how can it be solved?
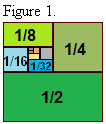
To rephrase the paradox, to be able to reach the tortoise, Achilles must first cover half the distance between himself and his goal. But before he can cover half the distance, he must cover a quarter of the distance and before the quarter, an eighth and so on. According to this interpretation, any motion is impossible. Achilles wouldn’t be able to take his first step, as there would be a smaller distance he would have to cover first. Using calculus as a way to wrap our heads around this problem, and supposing that Achilles can take his first step, if we add up all of the distances that Achilles must travel to reach the tortoise, we can represent it with what is called a geometric series:
This sum, visually represented in Figure 1, will add up to one (one being the area of the entire square), meaning that Achilles after some time has passed, will catch the tortoise. While some people believe that this solves the paradox and shows that Achilles catches the tortoise, many are not convinced. Using the calculus based mathematical solution we have had to modify the original problem by assuming that our hero can take his first step (and that there is not some distance he must first cover before taking said step). Since this is a modification of the original problem as defined by Zeno, many mathematicians consider the original rigorous definition of the paradox unsolved.
Modern versions
Though commonly referred to as Achilles and the tortoise, over the years many different names or variations have been found to represent the same fundamental paradox. Examples are “a student can never finish a test” and “a bullet can’t catch a running man”.2
Other types of paradoxical problems
When is a paradox not a paradox?
Let A equal B such that A and B are any two non-zero quantities
A = B
Multiply both sides by A
A2 = AB
Subtract B2 from both sides
A2 - B2 = AB-B2
Factor
(A-B)(A+B) = B (A-B)
Divide both sides by (A - B)
A+B = B
Subtract B from both sides
A = 0
If A was = 1, this shows that 1 = 0.
Thus A being a nonzero number at the beginning of the calculations is shown to equal zero. Can you locate the fallacy in this argument?
The problem with this proof occurs when dividing the equation by (A-B). The two quantities A and B are set equal at the beginning of the proof, so
(A - B) = 0, and therefore since division by zero is considered undefined in such situations, we cannot divide by it.
Another type of paradox is a flaw in a mathematical proof such as that in Figure 2.3 On first inspection, the proof may seem feasible. However, a hard to detect error hidden in the proof ultimately makes the statement false. The paradox arises because the statement seems sound, and if the reader is unable to locate the false statement, the proof reaches a seemingly absurd, un-mathematical yet intuitive conclusion.
The blank page
In some documents, such as manuals and exam papers, there exist pages that are left blank as placeholders or as by-products of the printing process. To avoid confusing the reader, who might think that the blank pages are printing mistakes, the publisher will add the following statement: "This page intentionally left blank". In this situation, the statement falsifies itself simply by existing. A blank page with writing on it is clearly not blank. Example of a “blank” page: [url=http://www.lichteblau.com/]http://www.lichteblau.com/[/url]
Twisted logic
"In the beginning there was nothing… which was a paradox because of its own existence, so it exploded.” -Douglas Adams
Like a security loophole in a software program, our own system of logic is vulnerable to being tricked, manipulated and misdirected. Paradoxes are designed to exploit these vulnerabilities. Over the course of history, humans have been able to come up with ideas or thought processes which present problems that defies our intuition. These are not flaws in our system of logic, but rather the end result of a series of steps designed to circumvent our natural thinking process and slip in a seemingly true statement with apparently sound logic. Paradoxes are a way of pulling a logical slight-of-hand, outwitting the reader using misdirection to the fullest to see if our inherent systems of logic can follow the presented idea.
Further reading
This site includes many more examples and explanations of common and obscure paradoxes: [url=http://www.paradoxes.co.uk/]http://www.paradoxes.co.uk/[/url]
Or if you prefer a physical book on the subject, try:
Van Orman Quine, Willard “The Ways of Paradox", The Ways of Paradox and Other Essays. Random House 1966. Rescher, Nicholas.
Paradoxes: Their Roots, Range, and Resolution. Open Court 2001.
Works Cited
1. Merriam-Webster Online Dictionary. "Contradiction" [url=http://m-w.com/dictionary/contradiction]http://m-w.com/dictionary/contradiction[/url]
2. Russell, Eric Frank. “Diabologic” Isaac Asimov's Wonderful Worlds of Science Fiction # 1: Intergalactic Empires. NAL/Signet 1983.
3. Mathematical proof inspired by [url=http://en.wikipedia.org/wiki/False_proof#Proof_that_2_.3D_1]http://en.wikipedia.org/wiki/False_proof#Proof_that_2_.3D_1[/url]
Thanks to Matthew de Courcey-Ireland, Andrew Katz, and Maria Bernier for their help with this article.

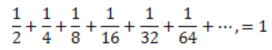
Comments
arielle
March 23, 2009This article is interesting in which it explains certain everyday things to be false, by proving so, when really most people who look at these falsities would think they are true. Nevertheless, with the example for the Achilles and the tortoise, the fact that the square will eventually be filled to the entire area is false because the squares inside will keep dividing; there is never a point where it stops even if it becomes too small to see that it doesn’t stop. This basically means that the Achilles will never catch up or pass the tortoise because the amount that is splitting each time he runs more and more will keep dividing never allowing him to actually reach the tortoise.
As for the article itself, it is well written and is not in essay-format which is obviously the goal and it was successfully reached. The examples given of everyday life together with the layout of the page makes the article that much more interesting.
You have to be registered and logged in in order to post comments!